Previous - Algebra 1: Algebraic Expressions and Indices

Solving for Variables

Solving for variables in equations require isolating these variables.

Example

(i) x – 6 = 0

To solve for x requires that we remove the 6 in order to have the x alone on the left hand side of the equation.  Because 6 is a negative 6, this means that we add 6 on both sides of the equation so that 6 alone remains on the left of the equation.  This will result in:

x = 6

(ii) 4x –  8 = 0

To solve for x requires that we first deal with the addition and subtraction.  This means that we must remove the 8 first.  Because the 8 is negative, we need to add a positive 8 on both sides of the equation to cancel it out on the left side and bring it to the right of the equation.  This leaves us with:

4x =  8

The next step is the multiplication or division part.  Because it is 4 multiply by x and it is positive, we need to do the opposite of multiply which is divide.  So we divide the 4x by 4 to leave the x on one side of the equation and we also divide the 8 on the other side of the equation by 4.  This leaves us with an answer of:

x = 2

(iii) 5x - 6 = 3x - 8        

Again, we first deal with the addition and subtraction.  In this example, we need to combine the likes such as the variables and the constants.  This means that we must combine the negative 6 with the negative 8 or have the 6 and 8 on the right side of the equation.  We do this by adding 6 to the left side of the equation and also to the right side of the equation.  The result will be:

5x = 3x -2

We next do the other part of the addition and subtraction so we must minus the 3x on the right side of the equation and also to the left side of the equation leaving us with

2x = -2.

We now turn to the division part and divide the 2x by 2 to isolate the x and also divide the -2 by 2 on the right side of the equation leaving us with the result of:

x = -1.

(iv)   3x + 1 = 4 
         4     2

In this equation, we have fractions and to add or minus fractions, we must find the least common multiple (LCM).  The LCM can be found by looking for the least common multiple of both denominators which are 4 and 2.  These common multiples of 4 and 2 are:

4: (4,8,12, 16,20,24,28, 32…..)

2: (2,4,6,8,10,12,14,16,18,20,22,24,26,28…..)

The LCM is 4 as this is the lowest multiple that is common to both 4 and 2. 

After manipulation, we get:

3x + 2  = 16
    4

x = 14/3 = 4 2/3

To solve for x in such an expression, we leave out the LCM to get:

3x + 2 = 16

So:

3x = 14

And x = 14/3 = 4 2/3.

(v)

      √ x - 9   = 4

The problem requires that we remove the square root sign on the left side of the equation or we times the expression by itself so:

      √ x - 9    times      √ x - 9    becomes x – 9.

Therefore, to remove the square root sign, we must time the expression by itself and do the same on the right side of the equation resulting in:

4²

So we now have:

x – 9 = 4²

x -  9     = 16

so x = 25

(vi) |4x - 1| = 7

This problem requires that we solve for an absolute number.  An absolute number is one that is within vertical bars such as above.  To solve for an absolute number, we need to remove the bars and make the number (or in this case, expression)  positive.  So this results in the following:

4x – 1 = 7

4x = 8

x = 2

Simultaneous Linear Equations

Simultaneous equations are two or more equations which have the same unknown variables and solutions. They are solved by using one of two methods: the elimination method or the substitution method. 

Elimination

In this method, the first objective is to eliminate one of the two unknowns (variables). This can be done by:

Adding the equations – this procedure is carried out if the coefficients of one of the unknowns are the same but have different signs.

Subtracting the equations – this procedure is done if the coefficients of one of the unknowns are the same and have the same sign.

If the coefficients of one of the unknowns are not the same, we can multiply one or both equations by a number(s) which will make the coefficients of the unknowns the same. Then, add or subtract the equations (depending on if they satisfy #1 or #2 above).

Example 

Solve simultaneously for x and y.

3x + y  = 5

x   -  y  = -1

Solution

In this case, in both equations, the y variables are the same with the same value of 1 for the coefficients for both equations but with opposite signs.  So we can simply add these two equations to eliminate the y variable.  This leaves us with: (3x + x) = (5 – 1) = 4x + 4) because the y variable was eliminated.

We can now solve for x by setting 4x + 4 = 0 and isolating x so that:

x = 1.

To solve for y, we can simply place the  x = 1 where x appears in either of the two equations. 

For equation 1:

3(1) + y = 5

3 + y = 5

y = 2

For equation 2:

We can also test this solution by substituting the x = 1 into the second equation so:

1 – y = -1

-y = -2

So y = 2.

So:

x = 1

y = 2.

Substitution

The following are the steps using the substitution method when solving simultaneous equations.

Step 1: Make one of the unknowns the subject of that equation or have it on the left side of the equation.

Step 2: Then substitute the value of the unknown above in the other equation and solve for the value of the other or second unknown.

Step 3: Using the solution for the second unknown, solve for the first unknown.

Solve simultaneously for x and y.

3x + y = 5

x   - y  = -1

Solution

Let us solve equation 1) for y which will give us a result of:

y =  5 – 3x and substitute this into the second equation.  We simply brought the 3x on the right side of the equation.

So we now substitute this y = 5 – 3x into the second equation to get:

x – (5 – 3x) = -1

x -5 + 3x = -1

4x – 5 = -1

4x = 4

x = 1

We can now insert this x = 1 value into equation 2 to solve for y such as:

1 – y = -1

-y = -2

y = 2

Note the same solution (x = 1 and y = 2) was obtained for both x and y using either the elimination or substitution methods.

Solving Simultaneous Equations with Three Equations and Three Unknowns:

Most equations that require simultaneous solution involve two equations and also two variables.  However, there are cases where there are three variables or unknowns and three equations.

Example

Solve this system of three equations with three unknowns:

1. 2x + y -  z  =  1
2. 6x + y + z  = -2
3. x   + y + z  =  3

Step 1: Reduce this to two equations in two unknowns.  This can be done by eliminating one of the unknowns from two pairs of equations: either from equations 1) and 2), or 1) and 3),  or 2) and 3)  Let us choose to eliminate z which can be done simply by adding equations 1) and 3) because the z variable in both of these equations has the same coefficient which is one (1) but opposite signs.  This will produce the following:

4)   3x + 2y = 4

Step 2: We now eliminate z from equations 1) and 2) which can be done by adding equations 1 and 2 to produce:

2x + y -z = 1

6x + y +z = -2

5)   8x + 2y = -1

Step 3: We then solve the two resulting equations which are equations 4 and 5:

4)     3x + 2y = 4

5)     8x + 2y = -1

This can be done by multiplying equation 4) by -1 to eliminate y.  The coefficients for the y variable will now be the same but with opposite signs such as:

-3x - 2y = -4

8x + 2y = -1

5x = -5

x= -1

So x = -1.

To solve for y, let us substitute  x = -1  into

3x + 2y  =  4 to get:

3(-1) + 2y = 4

or

-3 + 2y = 4

2y=7

y = 7/2.

Finally, to solve for z, substitute these values of x and y in one of the original equations such as equation 1 to get:

2(-1) + 7/2 – z = 1

-2 + 7/2 – z = 1

-4 + 7 – z = 2

-z = -1

z = 1

Solving Simultaneously with Quadratic Equations

Aquadratic is a type of problem that deals with a variable multiplied by itself — an operation known as squaring.  Quadratics is intrinsically related to a U-shaped curve known as a parabola.

Example

y = x² - 5x + 15

y = 3x - 1

Solution

Step1: Set both equations equal to each other.  Because both equations are already expressed in terms of y, we simply set both equations equal to each other such as follows:

x² - 5x  + 15 = 3x - 1

The aim is to solve for x.

Step 2: Subtract 3x from both sides of the equation to get: x² - 8x + 15 = -1

Step 3: Add 1 to both sides of the equation to get: x² - 8x + 16 = 0

 Step 4: Then solve the quadratic equation!

Start with: x² - 8x + 16 = 0

This can be done by using the FOIL method which will produce:

(x - 4 )   (x - 4)

So x = 4.

Step 5: Substitute x = 4 into both equations to get corresponding y values.

The matching y values are:

• y = (4)² – 5(4) + 15 = 16 – 20  +15 = 11

• y = 3(4) - 1 = 11

 Our solution: the two points are (4, 11) and (4, 11).

Solving for Quadratic Equations

Method: FOIL Method

F – first

I – inner

O – outer

L – last

Example

Solve for

x² + 12x + 27

Solution

First draw two open brackets such as follows
(x  +   9 )   (x  +   3 ).

First (F) in this case will be the 1^st two xs in both brackets as above.  The next step is the L for last which in this case will be any two numbers that when multiplied will equal 27 and when added or subtracted will result in a positive 12x for the middle number.   These numbers can be 9 times 3 = 27.  This positive 12x in the middle will be the sum of the I for inner and the O for outer such as: 9x + 3x = 12x.   To produce a positive 12x, both 9 and 3 must be positive. 

These two numbers look like 9 x 3 as follows:

(x  +   9 )   (x  +   3 )

The solution requires that we set x + 9 = 0 and when solving for x will produce x = -9

and x + 3 = 0 and when solving for x will produce x = -3

Example

x² + 8x + 7

Solution

The F for first will be x times x in both brackets such as:

(x  +  7) (x   +   1)

The L for last will be two numbers that when multiplied will result in a positive 7 and when added will result a positive 8x for the middle term.  This positive 8x will be the sum of the I for inner and the O for outer.  To produce a positive 8x, both 7 and 1 must be positive.  

So x + 7 = 0 so x=-7 and

x + 1 = 0

so x = -1.

Sum of Squares Method

Procedure for solving quadratic equations using the sum of squares method:

The quadratic equation is: ax²  + bx + c = 0

1. If the “a” does not equal 1, divide each side by a so that the coefficient of x² equals 1.
2. Move the constant term to the right.
3. Complete the square by adding the square of one-half of the coefficient half of x to both sides.
4. Write the left side of the equation as a square and simplify the right side of the equation.
5. Then equate and solve for x.

Example

Solve the following equation:

2x² + 8x - 16

Solution

Because “a” in the equation is not 1, we need to divide by 2 throughout to make it 1 so the equation now becomes:

x² + 4x - 8

then we move the 5 to the right side of the equation by adding it to both sides of the equation to result in:

x² + 4x = 8.

Next we need to take one-half the coefficient of the “x”.  Therefore, to complete the sum of squares, we need to take half of the coefficient of “x” and square it. The coefficient of x is 4 and 1/ 2 of this is 2.  Squaring this will result in 4.

The next step will be to add this result to the right of the 4x on the left side of the equation and also to the right side of the equation which will result in:

x² + 4x + 4= 12

The next step will be to rewrite the above as a square such as:

(x + 2)² = 12

x + 2  = -2 + √ 12

Quadratic Formula Method

The Quadratic Formula requires we have the quadratic expression on one side of the equal sign, with zero on the other side. The formula is also stated in terms of the numerical coefficients of the terms of the quadratic expression. The quadratic equation is given in the following form:

ax² + bx + c = 0

Example

Solve for x in the following equation

x² + 4x - 8

Solution

Looking at the coefficients in this equation: a = 1, b = 4, and c = –8. I'll plug these numbers into the Formula, and simplify. (I should get the same answer as I previously have.)

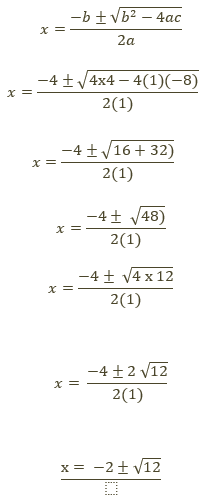
Note that the same solution using the sum of squares method was also obtained using the quadratic formula method.

Linear Inequality

All the rules governing the solution of linear equations apply to the solution of linear inequalities, except the following differences:

1. Inequalities do not have equal signs. They are represented by the signs:  < which means, less than; ≤ which means, less than or equal to; > which means, greater than; ≥ which means, greater than or equal to.
2. When an inequality is multiplied or divided by a negative number the sign changes. That is, a < (less than) sign would change to a > (greater than) sign, a ≥ (greater than or equal to) sign would change to a ≤ (less than or equal to) sign.
3. The solution of an inequality is a range, which can be drawn on a number line, and is therefore written as a solution set.

Example

Find the solution set for x > -1 and x < 3 which can also be written as -1 < x < 3.  This means that x can only fall between -1 and 3.

Solution

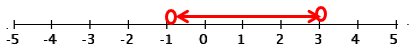
The line shows empty circles which means that -1 and 3 are not included. 

The graph of an inequality with an "or" represents the union of the graphs of the inequalities. A number is a solution to the compound inequality if the number is a solution to at least one of the inequalities. It is written as x < -1 or x > 1.  The line shows an empty circle which means that -1 and 1 are not included.

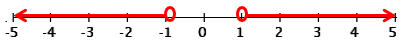
Example

x ≥ -2

Solution

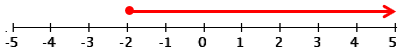
The line shows a full circle which means that -2 is included.

Inequalities

Solving inequalities is not so different from solving regular equations. In fact, an inequality sign (<, >,≤,, ≥) is treated the same as an equal (=) sign when solving inequalities involving only addition or subtraction. Let us define the different inequality signs:

< indicates that the expression on the left is less than the expression on the right, for example:

2 < 4

indicates that 2 is less than 4.   

7 > 4

shows that 7 is greater than 4 and similarly

4x – 8 > 11

means that the value of 4x – 8 is greater than 11

Solving Inequalities

Most linear inequalities can be solved just the same as linear equations: Addition and subtraction of any number (positive or negative) can be done to the expression on either side of the inequality without changing the inequality itself. In other words, it would be the same as in any ordinary equation.

For example: Solve for x in

x + 8 > 12

Solution:

Subtract 8 from both sides of the inequality as follows:

x + 8 – 8 > 12 – 8 to get

x > 4

Observe that the above is solved in the same was as solving ordinary equations. This is because addition and subtraction have no effect on the inequality sign. Multiplication and division are different, however, as the inequality sign is treated differently depending on whether we are multiplying by a positive or negative number.

Multiplying or dividing through the inequality expression by a positive number has no effect on the inequality sign and is treated as you would to an ordinary equation.

For example: Solve for x in:

5x < 25

Solution:

First divide both sides of the equation by 5 to get:

x < 5.

On the other hand, when multiplying or dividing through the inequality by a negative number, this has the effect of reversing the inequality sign, for example from < to > as shown below

-4 – 2x < 8

is solved as follows;

adding the 4 to both sides to get:

-2x < 12 then

Dividing by -2 reverses the inequality sign resulting in:

x > -6.

This means that x can take on any value as long as that value is greater than -6.

Next - Geometry 1: Types and Properties of Angles and Triangles